2ndUS/China/Japan Conference of Physics Educationü@
Fuji, Japan 18-22 July 1991
The Article carried in ügIKIIKI WAKUWAKUüh editor George Marx, OOK-PRESS
JAPANESE-HUNGARIAN PHYSICS TEACHER MEETINGü@
Jászberény, Hungary, 18-22 August 1992
HOW TO FORM A SCIENTIFIC CONCEPT?
Yoji IIDA
Nagoya Tomida High School, Nagoya, Japan
What is necessary to develop a scientific concept?
Concepts and laws are by nature abstract, essential and invisible. They are difficult to understand through direct experience or feeling. So, the duty of education is to make something invisible to a visible one. it is very important to mediate phenomena and their essence by substantialistic models:
-Simple model of the structure of an object.
-Spring model of force.
-Atomism as a view of nature, -and some philosophy added.
M. Taketaniüfs three stages of recognition are (1936): Phenomenological –substantialistic -essentialistic stages. His theory is said to have been the philosophical and methodological basis of Yukawa's meson theory. This theory of cognition is very effective to predict unknown phenomena and laws.
Taketani's theory was applied to a practical teaching, leading to the ügHypothesis -Experiment -Instruction Method" of K. Itakura(1963). What is known to scientists is unknown to students. Through Itakura's method, students succeed in finding a law in the same way as scientists do. There are three stages of prediction, according to K. Shoji, (1976):
1. Phenomenological stage: ügSomehow, perhaps, probably, according to my intuition, as I guess, etc. (Simple, individual, concrete and sensitive.)
2. Substantialistic stage: ügFor example, similarly, analogically, through experience, by metaphorical image.üh (Middle of phenomena and essence, peculiar, half -abstract, half-logical.)
3. Essentialistic stage: Prediction based on a law, reasoning, hypothesis, (Universal, general, abstract, logical and reasonable.)
4. Ups and downs of student's recognition: Students go through these three different stages of recognition through their discussion, and move from one stage to another.
Only the stage 3 has been approved for education and the stages 1. and 2. have been disregarded. I think that the stage 2 is particularly important in order to reach stage3.
How do students succeed in using a scientific concept effectively?
My current studies and practices have developed from the spirit of Itakura's method. For students to fix scientific recognition in their mind, I think, at least the following steps should be taken:
(1) Give questions in which issues are clear!
Each question should make a striking contrast between the logic of science and that of common sense. In order to construct those questions, it is necessary to analyze the fundamental concepts of physics (to check their meaning and range of validity) from the teacher's viewpoint and, at the same time, to grasp students' preconceptions (their judgments on the basis of everyday experiences).
(2) First let students predict, and then discuss!
Each student should set forth predictions or hypotheses concerning presented questions, explain why he or she does so, write down his (her) views, and discuss the reasons. While students are doing so, their ways of thinking based on their experiences may be developed. Even if they are wrong from a teacher's viewpoint, we must not try to suppress their ways of thinking. This is a very good chance to grasp their preconceptions. They should make a discussion thoroughly following their own logic.
(3) Perform an experiment!
Which prediction or hypothesis is correct is not declared by the authority of a teacher but judged by an experiment. The simpler the experiment is, the more clearly its essence can be seen. With their heart beating in excitement, anyone can not help watching the process of an experiment because their predictions or hypotheses are tested. There is no doubt that they shout for joy, whether the result is as expected or not. Here is a secret why they exclaim, ügFantastic!" or ügInteresting!"
(4) Pile up questions and experiments!
The presentation of questions and experiments enables students to overcome their views on nature supported by everyday experiences, and to recognize that
judgment based on logic of science is much more valid than judgment based on
everyday experiences.
(A) The concept of speed
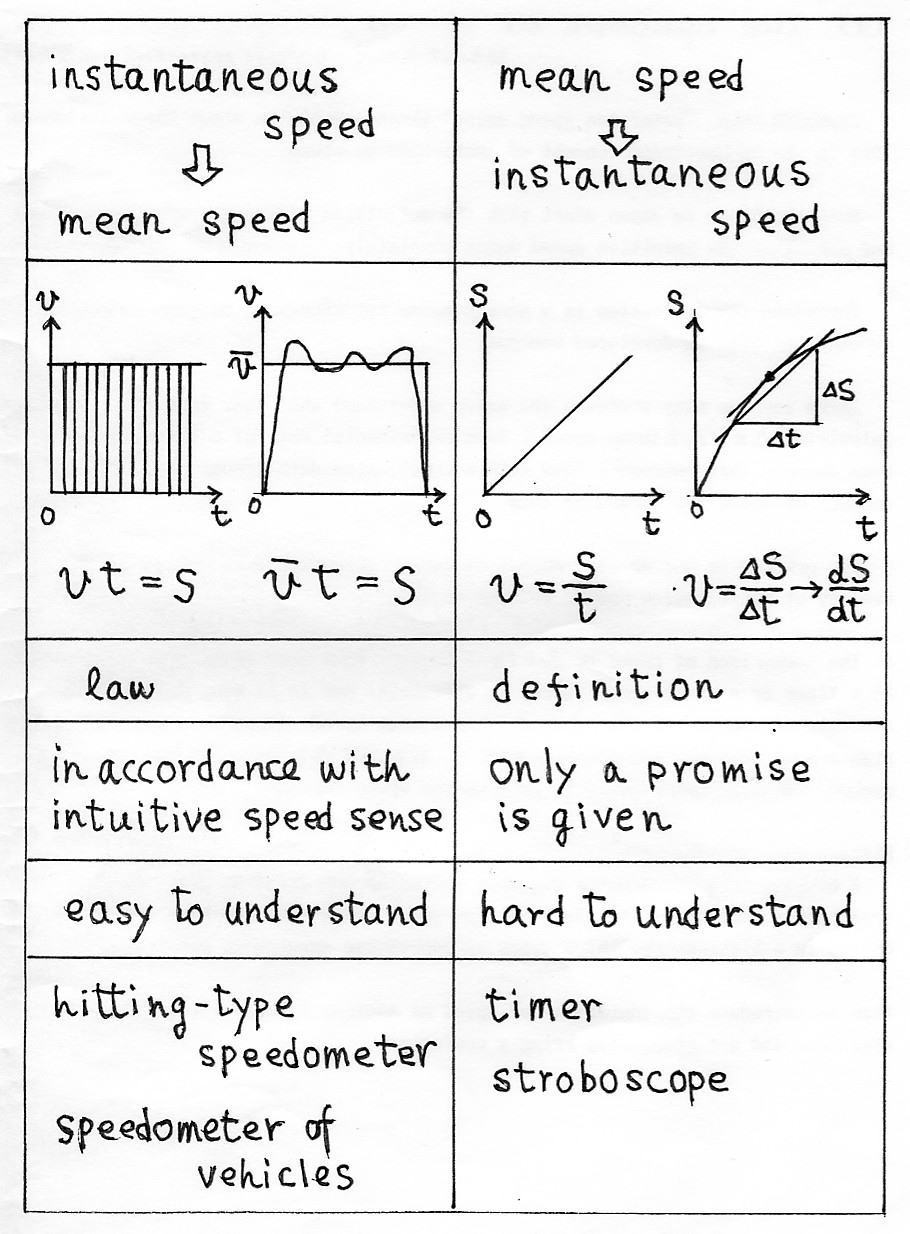 Students have got ügintuitive sense of speed" through their experiences in vehicles since their childhood. The undeveloped concept is that of the instantaneous speed. Most textbooks in Japan start with the definition of speed v=s/t (mean speed) and disregard the
intuitive speed sense completely. Therefore the definition
is a mere promise for students because they have already had this undeveloped
concept. There were many students who never understood what they were doing
when they calculated çÖéô/çÖéö(mean speed ) from experimental data of a timer (diffused
all over Japan). This impressed them with a complicated data processing, but no
concept of speed was formed by them. The problem is how we can develop
students' intuitive speed sense of speed to the concept of quantitative speed,
relying on them. The comparison of speed by çÖéö(if s=const.) in a race or by çÖéôüiif t=const.) in a timer
or a stroboscope is not so difficult. But it is more difficult for students to
understand that çÖs /çÖt is a mean speed. Japanese junior
and senior high school students calculate çÖs /çÖt from çÖt and çÖs by using the operating method, ügHajikiüh rather than by forming the
speed concept.
Students have got ügintuitive sense of speed" through their experiences in vehicles since their childhood. The undeveloped concept is that of the instantaneous speed. Most textbooks in Japan start with the definition of speed v=s/t (mean speed) and disregard the
intuitive speed sense completely. Therefore the definition
is a mere promise for students because they have already had this undeveloped
concept. There were many students who never understood what they were doing
when they calculated çÖéô/çÖéö(mean speed ) from experimental data of a timer (diffused
all over Japan). This impressed them with a complicated data processing, but no
concept of speed was formed by them. The problem is how we can develop
students' intuitive speed sense of speed to the concept of quantitative speed,
relying on them. The comparison of speed by çÖéö(if s=const.) in a race or by çÖéôüiif t=const.) in a timer
or a stroboscope is not so difficult. But it is more difficult for students to
understand that çÖs /çÖt is a mean speed. Japanese junior
and senior high school students calculate çÖs /çÖt from çÖt and çÖs by using the operating method, ügHajikiüh rather than by forming the
speed concept.
Hitting-type speedometer:(click)
A hitting-type speedometer compares speeds by hitting. So this speedometer corresponds to the intuitive sense of speed the students have. Moreover the device is a hitting-type which makes an impressive experiment possible. When we introduce the concept of instantaneous speed, as question 2, only v-t graph is used. A s-t graph would bring a confusion.
The question which shows a striking contrast between the logic of science and that of common sense.
üyQues. A-1üz
Which is faster, A or B at point P?(click)
Prediction:
(a) VA üä VB
(b) VA üü VB
(c) VA üâ VB
Discussion:
(c) A steep slope accelerate the ball sharply.
(a) If the ball is accelerated in a long distance, it goes so much the faster.
(b) If the above-mentioned opinions cancel each other, A and B have the same speed.
Experiment: hitting-type speedometer
Accumulation of predictions, discussions and experiments for some related questions.
By inspecting each of their hypotheses, they obtain the effectiveness of scientific logic. (the omission of examples)
üyQuestion A-2üz
While the Shinkansen Hikari from Nagoya to Tokyo was running, its speedometer recorded a speed at an interval of a minute shown in the follwoing graph.
Predict approximately how long the train ran for two hours.

I predict it is about ( ) km.
Hint: How long it will run at a constant speed of 170 or 200 km/h.
Inspection:It is ( ) km from Nagoya to Tokyo by Shinkansen according to
materials from the JR. (342.1km)
Does the answer meet your prediction?
A slope is a tool to delay time.-Toy balloon hovercraft-
üyQues. A-3üz
A gentle slope. How will a glass ball gain speed?
If a gradient is increased a little, what will happen?
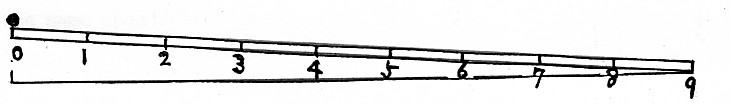
Prediction:
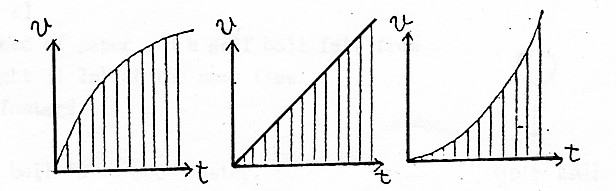
Experiment:
We don't need a watch to measure time. The use of a slope allows us to delay as much time as we want.
Everytime a glass ball passes point 1, we put another glass ball at point 0 and release it. So a regular interval of time is attainable. If balls falling at such regular intervals of time line up at points 1, 4, 9 at the same time, this is the proof of uniformly accelerated motion.
üyQuestion A-4üz
(1) When a ball falls from the point l on a gentle slope, it hits the cap X.
From what point should it be dropped to gain a doubled speed (to hit the cap Y) ?
Predictisn: a. point
2
b. point 4 c. point
1.4
d. others
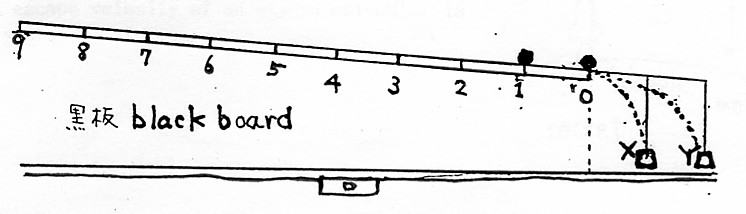
You should check if the ball will hit the cap when it is dropped from each of the predicted point.
(2) From what point should the ball be dropped to gain a threefold speed?
(3) Repeat the same experiments of (1) to (2) by changing a slope a little.
Remark on Ancient Atomism
For the motion of rain drops the Aristotelian physics is correct. Students in a school which is said to be good in Japan are apt to answer on the base what they have learned rather than to observe an actual motion.
In a vacuum both a feather and a piece of metal fall on the Earth in quite the same time, irrespectively of mass. So this is a universal law applicable to any mass. Everything comes down at the same time as a pebble does whether it may be as tiny as an atom or as large as the moon. Students, however, never think that the escape velocities of a hydrogen molecule, a pebble and a rocket are the same.
According to the ancient atomism there was nothing in an empty space to obstruct any motion, which showed no variation and it was not affected by mass. (We can tell the difference of mass by obstructing a motion). This is an inertial
motion by itself. Galileo applied this to the free fall regardless of the cause of acceleration. It becomes clear that the difference of mass has no effect on the free fall as far as no obstacle exists. Vacuum is an empty space in which there is no atom and no resistance to any motion at all. So it is a dynamical vacuum. The concept of space in Newtonian dynamics is a uniform space. The dynamical vacuum of the atomists is very significant to form the concept of space.
In the Ancient Atomism
Atom: invariability of substance, and obstruction of another atomic motion, (the idea of indestructibility of matter)
Vacuum: guarantee of the motion of substance, and not an obstruction of any motion, (the idea of indestructibility of motion).
No resistance to motion at all means a dynamical vacuum. As far as no obstacle exists, it is natural that a body continues its motion. Atomism as the view of nature is a natural base of dynamics such as the law of inertia and the law of conservation of momentum are.
üyQuestion A-5üz
Which fall faster to the ground, larger or smaller drops of rain?
Prediction:
a. Larger drops.
b. Smaller drops.
c. The same speed.
d. Dependent on time and occasion.
üyQuestion A-6üz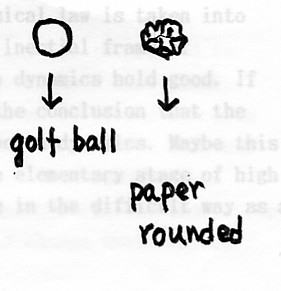
A rounded piece of paper and a golf ball fall from the same height (1-2m) at the same time.
Which falls faster?
Prediction:
a. A golf ball falls much faster.
b. A golf ball falls slightly faster.
c. A rounded piece of paper falls slightly faster.
d. With almost the same time.
(We can hardly tell the difference.)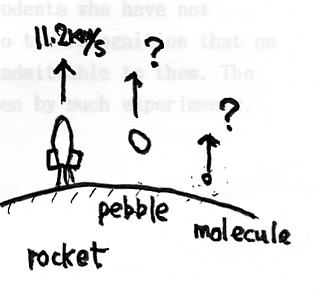
üyQuestion A-7üz
The escape velocity of a rocket from the earth is 11.2km/s.
If the following senteces are correct, draw a circle or if not, put a cross.
(1) The escape velocity of a pebble is 11.2 km/s.
(2) The escape velocity of an oxygen molecular is 11.2km/s.
(B) The concept of Inertia
How to overcome studentüfs concept of üga moving force"?
Students should experience a world where an object maintains its motion when no force is acting on it. We introduce Galileoüfs principle of relativity as an actual premise. A ball dropped on the running vehicle keeps moving in the forward direction together with the vehicle.
Students' previous judgment clash with Galileo's principle of relativity that a state of uniform motion is quite equivalent dynamically to the state of rest. Students are driven to alter their judgment fundamentally. The recognition of the dynamical equivalence of rest and uniform motion -the principle of relativity- gives a true account of meaning that feeling and experience are not reliable. The Earth is moving but we cannot feel it. This can be interpreted as a wonder, but the principle of relativity is a law which prohibits us from feeling motion.
The
question B-1 never fails to make students excited. A mere thought of this kind
of the question clashes with their own view of rest, and they will discuss the
problem, which answer is correct. In this stage they should discuss the problem
sufficiently, make an impressing experiment and recognize experimental facts. These
are starting points.
üyQues. B-1üz 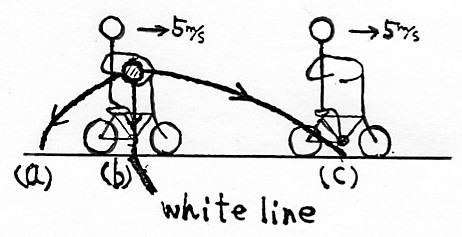
Where will a ball land on the ground, if it falls freely just above the white line?ü@
Prediction:
a. Back of the white line.ü@ü@ü@ü@ü@ü@ü@ü@ü@
ü@b. On the line, far back of the bicycle.
ü@c. Ahead of the line, at the same position as the bicycle.
ü@d. In other places.
Discussion:
a. I suppose a dropped piece of paper is fluttering behind the bicycle.
b. I'm sure it drops on the white line just below because any force does not act on it.
c. A ball continues moving together with the bicycle and can't stop its motion if it is released from the hand.
d. I'm sure it drops between (b) and (c) because air resistance acts on it.
Experiment:
Accumulation
of predictions, discussions and experiments for some related questions.
By inspecting each of their hypotheses, they obtain the effectiveness of scientific logic. (the omission of examples)
Why isn't the principle of relativity used in physics at high school?
I wonder that the principle of relativity can not be found in textbooks for high school students. It is important, and can be introduced in an exciting and easy way. Usually they study this principle only when they are college students. College textbooks used to say :
(1) The dynamical law does not change under the Galileo transformation.
(2) All the coordinate systems, in which the Newtonian dynamics is valid, are dynamically equivalent.
No. (1) is a description in which the symmetry of a physical law is taken into consideration. No. (2) is a description in which the inertial frame of reference is taken as a condition on which the Newtonian dynamics hold. If the descriptions are interpreted literally, we come to the conclusion that the
principle could not be taught before learning the Newtonian dynamics. Maybe this is why the principle is not taught in high school. But in the elementary stage of high school education there is no need to teach it in this difficult way!
(3) No dynamical difference can be found empirically between observations made in a state of uniform motion and in a state of rest (dynamical equivalence of a state of rest and a state of uniform motion).
It is not so difficult for students, who have not learned anything about Newtonian dynamics before, to jump up to the recognition that no dynamical difference exists among typical experiments performed in different frames of reference admittable to them. The problem is how we can show students such experiments.
(C) The concept of force
How to overcome a ügmoving forceüh?
üyQues. C-1üz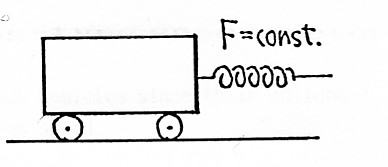
At what speed will the cart go?
Constant force. Low frictional force.
Prediction:
a. At constant speed.
b. Accelerating and then at constant speed.
c. Accelerating.
Discussion:
a. With constant force acting on the cart, it continues a state of constant speed.
b. Constant speed is not attainable immediately after it starts moving.
Think about a car for example. But it attains constant speed very soon.
c. Without any force acting on the car, it moves with constant speed. With force acting on it, it continues accelerating.
Experiment:
Accumulation
of some related questions:
First of all in the question C-1, students should confront themselves with a common sense view: Fü`v. The discussion is developed by three divided groups. A group develops their logic of the law of inertia: an object keeps its constant speed if a force doesn't act on it. If an impulse is added, it moves faster and reaches a larger constant speed. If it is added intermittently, it moves faster and faster. But this logic is not enough to overcome the other studentsüf Aristotelian ideas.
In the experiment related to C-1, a cart is drawn on the corridor. Students should discuss this question. The more exciting their discussion is, the more impressing the experiment will be. They come to have the recognition that the change of velocity corresponds to a constant force.
üyQues.C-2üz
Which
is larger, A or B?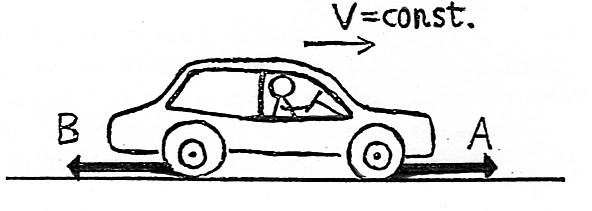
A; The forward force.
B; The backward force.
Predicton:
a. A<B
b. A=B
c. A>B
Discussion:
a. If the forward force is not greater, it cannot move. The balance of A and B causes it to stop its motion.
b. If the forward force is greater, it will continue accelerating.
c. If the backward force resisting a forward motion is greater,
it will move unif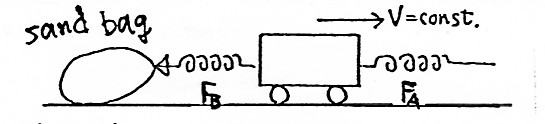 ormly.
ormly.
Experiment:
Accumulation
of some related questions: (the omission of examples)
During the discussion of the question C-2, there is a striking confrontation between those who try to develop their logic, and others who try to stick to their own earlier feeling. Based on the question C-1 some students emphasize : The more force acts, the faster an object moves. On the contrary others stick to their previous feeling and refute their opponent by saying that if forces are balanced, an object will not move. Then a student adds his opinion: ügWe have learned that a state of uniform motion is the same as a state of rest. In the case of uniform motion the forces are balanced in the same way as at rest and a object should maintain its motion. This is what we call the law of inertia, isn't it?" This way of thinking does not appear at the majority but the development of it causes the groups to start thinking.
The experiment is designed to draw a cart with a sand bag at almost constant speed (which can be easily seen) and compare a stretching of the spring in front and at the back of the cart. This question is for the development from the law of inertia applied under the condition that no force is applied to the law of inertia applied under the condition of balanced forces. The questions C-1 and C-2 make a pair, they are both indispensable as key points.
There are still many students claiming that a force in the forward direction exists in the stage of the question C-3. Here they confirm the principle of relativity that the same force acts in uniform motion as at rest and that there is no dynamical distinction between a state of uniform motion and a state of rest. Additionally the force must be defined: What you call as a moving force is a the amount of motion a moving object has, isn't it? This may be called as momentum what weüfll learn later, not force. You are confused if you call it force. Force is not a volume what a substance has, but what is acted from outside. What changes the shape and movement of a substance should be called ügforce"
When the concept of force and the principle of relativity are introduced to students, these clash with un-developed concepts of force students have had. The un-developed concepts become developed concepts on the base of experimentation and discussion. When students get convinced of the law of inertia that the ügmoving forceüh is quite different from force, and an object moves without a force, they began to change their way of thinking very fast.
Students may think: As an object falls, gravity is increased or a falling force is added. They are still in the stage of the question C-5. A force acting on an object moving along a parabolic trajectory is still drawn in the movingü@direction by some students. In this stage all kinds of forces drawn by students are used on the blackboard and correct forces are selected through discussion, based on the principle of force and law of inertia. At last at this stage almost all students begin to get convinced.
[D] The concept of force to change direction
üyQues.D-5üz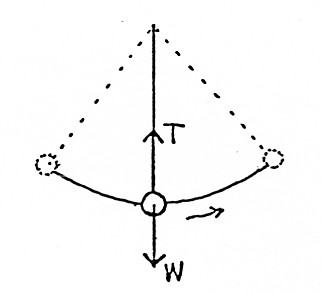
A swinging pendulum. At the lowest position.
Which is greater, tension T or gravity W?
Prediction:
a. T > W
b. T = W
c. T < W
Discussion:
a. You get on a swing and try to make an effort to swing at the lowest position, don't you?
b. The majority of college students answered that T and W are balanced.
c. If they are balanced, uniform linear motion is provided and curved motion can not be provided.
Accumulation of some related questions: (the omission of examples)
(E) Heat is equivalent to work
(1) Let's shake a thermos bottle.
-ügGee! Temperature is really rising!üh- students understand that work equals heat.
-ügI've swung the pot so many times, but it's risen only one degree!üh- students
understand that the energy value of heat is very different from that of work.
They are very surprised to find these facts through their direct experience.
(2) From dependence on mathematics to qualitative and conceptual understanding.
Quantitative pursuit doesn't always allow students to realize essential understanding. In the early stage qualitative or conceptual understanding prior to quantitative understanding is quite necessary. Today the education of physics is too much dependent on mathematics, which results in obstructing qualitative or conceptual understanding. Calculation pays a leading role at the entrance examination in physics. If questions asking for students' qualitative or conceptual understanding were made at the entrance examinations in Japan, a more smooth progress of high school education in physics could be carried out. Especially when students start to study physics, dependence on an algebraic formula is a big obstacle to the formation of a model concept. It is important to aim at qualitative or conceptual understanding without making use of math so often.
(3) The image of molecular motion.
From the beginning students don't have the image that a molecular motion is at random. The image can not be obtained through experience and is not formed naturally. If teachers show students this concept in their early stage, it is easy to understand mechanical equivalent of heat or energy conversion by using the image of random motion of molecules.
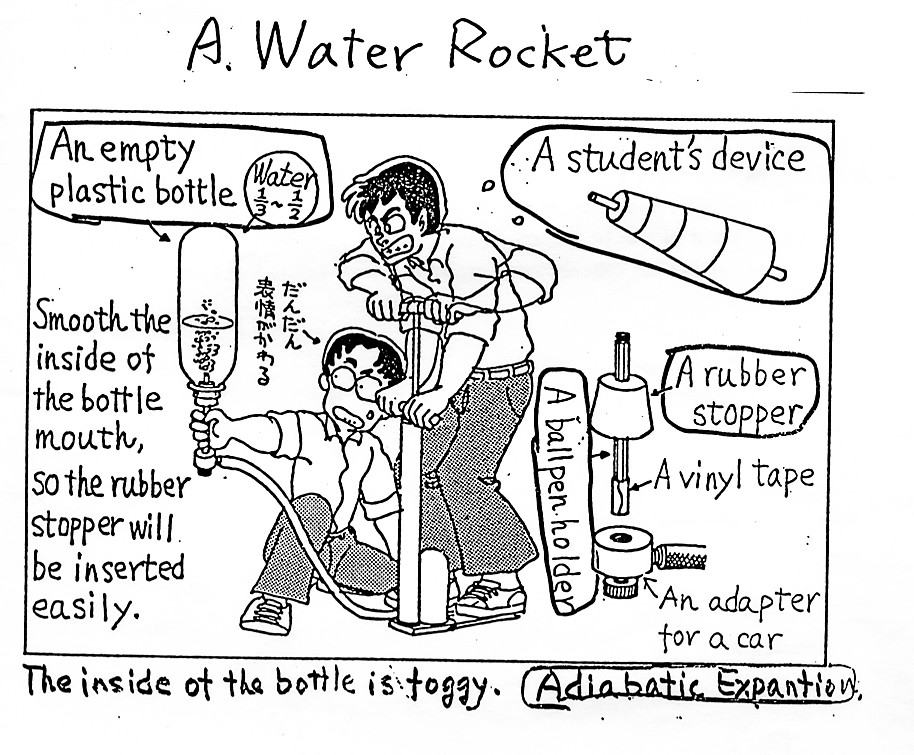
Adiabatic Expansion (air rocket)
Adiabatic Compression (click)
The image of molecular motion (click)
A Random Motion Cart (click)